Yet Another Simple Integral
Problem
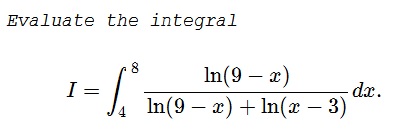
Solution 1
The integral falls into the category described earlier that we generalize a little below:
Let $a,b$ be two real numbers, $f$ an integrable function on a sufficiently large interval. Assume $f(x)+f(2a-x)=1$ for $x\in [a-b,a+b].$ Then
$\displaystyle \int_{a-b}^{a+b}f(x)dx=b.$
The assertion follows from the observation that, for any integrable function $f,$ $\displaystyle \int_{a-b}^{a+b}f(x)dx=\int_{a-b}^{a+b}f(2a-x)dx.$
Any number of examples of such functions $f$ can be produced from the formula $\displaystyle f(x)=\frac{g(x)}{g(x)+g(a-x)}$ and the function in the problem $\displaystyle f(x)=\frac{\ln(9-x)}{\ln (9-x)+\ln(x-3)}$ is of that kind: $g(x)=\ln(12-x),$ with $a=6.$ Indeed, the substitution $y=12-x$ yields
$\displaystyle \begin{align} f(12-x)&=\frac{\ln(9-(12-x))}{\ln (9-(12-x))+\ln((12-x)-3)}\\ &=\frac{\ln(x-3)}{\ln (x-3)+\ln(9-x)}\\ \end{align}$
so that $f(x)+f(12-x)=1.$
Since in the problem the integration is over $[4,8]=[6-2,6+2],$ $b=2$ and this is the value of the integral:
$\displaystyle I=\int_{4}^{8}\frac{\ln(9-x)}{\ln (9-x)+\ln(x-3)}dx=2.$
Solution 2
First make a substitution $x=y-6$ to obtain
(1)
$\displaystyle I=\int_{-2}^{2}\frac{\ln(3-y)dy}{\ln(3-y)+\ln(3+y)}.$
Now substitute $y=-u:$
$\displaystyle I=-\int_{2}^{-2}\frac{\ln(3+u)du}{\ln(3+u)+\ln(3-u)}.$
Interchanging the limits cancels the minus sign:
(2)
$\displaystyle I=\int_{-2}^{2}\frac{\ln(3+u)du}{\ln(3+u)+\ln(3-u)}.$
Replacing $u$ with $y$ and adding (1) and (2),
$\displaystyle 2I=\int_{-2}^{2}\frac{\ln(3-y)+\ln(3+y)dy}{\ln(3+y)+\ln(3-y)}=4.$
Illustration

Acknowledgment
The problem 997 from the Pi Mu Epsilon Journal (Fall 2001) was reproduced in Ross Honsberger's Mathematical Delights (MAA, 2004, p 78) with credits for the solution (Solution 2) to Sophie Trawalter.
- A simple integral, I
- A simple integral, II
- A simple integral, III
- Another simple integral
- Yet Another Simple Integral
- Integral in Normal Distribution
- Sum Without Adding
- An Integral Inequality from the RMM
- Another Integral Inequality from the RMM
- A Triple Integral Inequality
- An Integral from the RMM
- An Integral Identity with Two Functions
- A Simple Integral of a Peculiar Function
|Contact| |Front page| |Contents| |Algebra| |Up|
Copyright © 1996-2018 Alexander Bogomolny
71552613
